





A fine thing about minimal surfaces is that they are easily obtained in real-life. In fact, if you construct a closed curve with a wire, and then put it in a soap solution then you will get a soap film (a surface) wich has the wire as boundary. This surface is a minimal surface which solves Plateu's Problem.
What follows is a tracking shot of minimal surfaces.

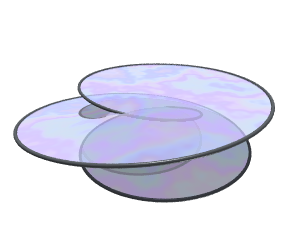
 For example, if we take two circles as boundary, we get two different local minimizers a disconnected one and a connected one: two disk or the cathenoid.
For example, if we take two circles as boundary, we get two different local minimizers a disconnected one and a connected one: two disk or the cathenoid.
Considering the following closed curve:


 we get two local area minimizers: a non orientable surface (the Möbius band) and
an orientable one.
Notice that both these surfaces are regular.
we get two local area minimizers: a non orientable surface (the Möbius band) and
an orientable one.
Notice that both these surfaces are regular.
You get an example of a singular minimal surface if you consider the following
boundary curve:

The global minimizer, in this case, is a surface which has a circumference of singular points (there are three surfaces joining in such points with an angle of 120 degrees):
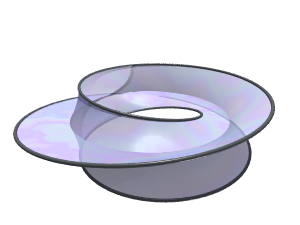 we get local minimers if we impose the surface to be regular (but not oriented):
we get local minimers if we impose the surface to be regular (but not oriented):
 or oriented:
or oriented:

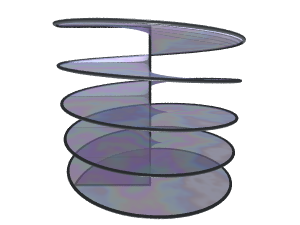
 In the following example we see a minimal surface with infinite
genus. Notice that the boundary is again a continuous closed curve.
In the following example we see a minimal surface with infinite
genus. Notice that the boundary is again a continuous closed curve.
To give a mathematical form to the Plateau's problem it is necessary to choose a good definition of what a surface is. A possibility is to define a surface as the image of a continuous map over a certain two-dimensional domain (parametric surface).
 For example this minimal surface (helicoid) can be
parameterized over a disk-type domain.
For example this minimal surface (helicoid) can be
parameterized over a disk-type domain.

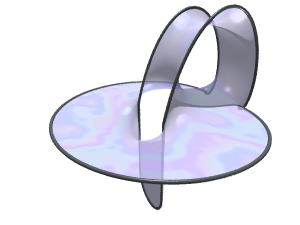 For example these surfaces have the same boundary.
The surface on the left is the least area surface among all parametric surfaces wich have a disk as base domain.
While to the right, we have the minimal surface that we obtain as a
soap film.
Note that the function describing the parametric surface is not injective (the
surface intersects itself) while the "true" minimal surface is regular in all points.
For example these surfaces have the same boundary.
The surface on the left is the least area surface among all parametric surfaces wich have a disk as base domain.
While to the right, we have the minimal surface that we obtain as a
soap film.
Note that the function describing the parametric surface is not injective (the
surface intersects itself) while the "true" minimal surface is regular in all points.
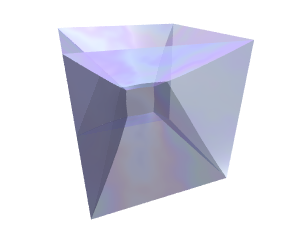 For example, this is the minimal surface with boundary the edges of a cube.
Such surface is also interesting because you can find both types of singular points that can be found in minimal surfaces.
In fact there are some points (along 12 segments) of the surface in which three foils meet (with an angle of 120 degrees) and there are four points (the vertices of the square in the center) where four foils meet with an angle of about 108 degrees.
For example, this is the minimal surface with boundary the edges of a cube.
Such surface is also interesting because you can find both types of singular points that can be found in minimal surfaces.
In fact there are some points (along 12 segments) of the surface in which three foils meet (with an angle of 120 degrees) and there are four points (the vertices of the square in the center) where four foils meet with an angle of about 108 degrees.
In this example we see a surface which retracts on its boundary.

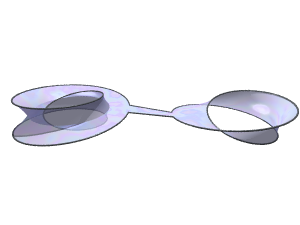 It is possible (but this is no way intuitive) to deform this surface, always letting the boundary fix, up to let it coincide with its boundary.
So, in a certain sense, this surface is not a global minimum of area since it may be deformed to a surface with null area.
It is possible (but this is no way intuitive) to deform this surface, always letting the boundary fix, up to let it coincide with its boundary.
So, in a certain sense, this surface is not a global minimum of area since it may be deformed to a surface with null area.

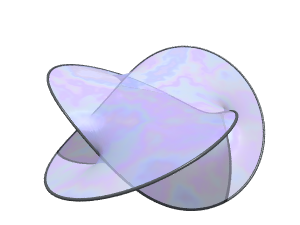
This example is maybe only a curiosity. We know that given any closed curve
it is possible to find a surface with least area which has this curve as boundary.
So, what is the shape of a surface which has as boundary a knotted curve?
In this example we see the minimal surface with boundary the trefoil knot,
which is the simplest, non trivial, knot.
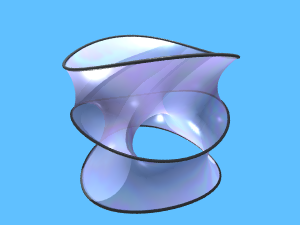 In the last example we see that it is not always obvious to understand what is
the shape of the minimal surface with a given boundary.
Here we consider as boundary the union of three disjoint circumferences.
The minimal surface obtained is a surface "omeomorphic" to a disk with two holes and a handle.
In the last example we see that it is not always obvious to understand what is
the shape of the minimal surface with a given boundary.
Here we consider as boundary the union of three disjoint circumferences.
The minimal surface obtained is a surface "omeomorphic" to a disk with two holes and a handle.